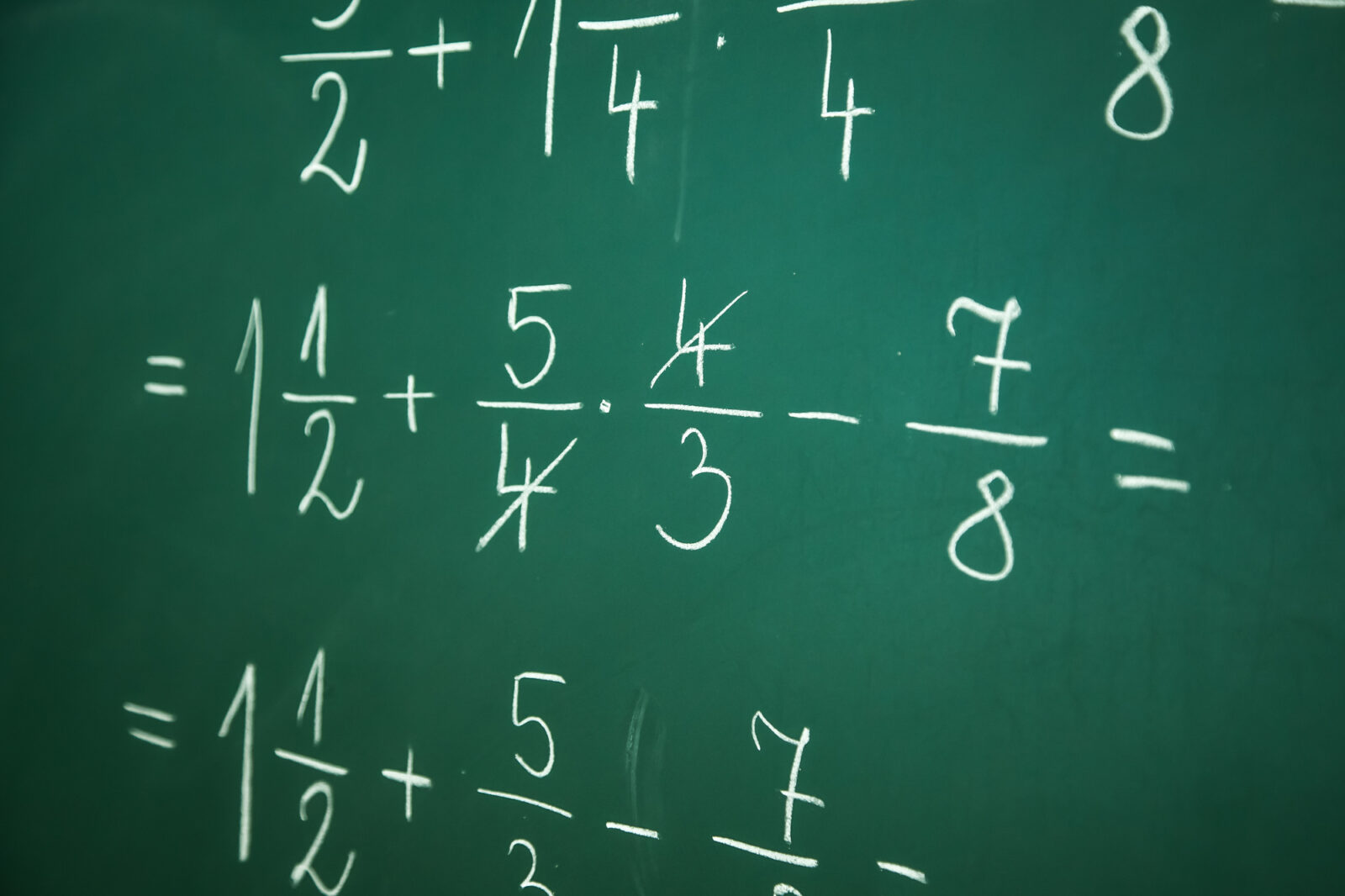We learn fractions from an early age, and the reason for that is simple: we use fractions in our daily lives, especially when we grow older. It has applications in cooking, shopping, and other daily functions.
Adding fractions or subtracting is still pretty simple, but fractions can be pretty confusing when you get to the multiplying and dividing.
Do you find it difficult? We’re here to help. Review the steps below to learn multiplying and dividing fractions easily.
Different Types of Fractions
Let’s refresh our memory, first. There are 3 kinds of fractions:
- proper
- improper
- mixed
The process on how to multiply and divide fractions is basically the same no matter what fraction you have, but there will be an extra step based on the type.
A proper fraction has a numerator (the number on top) that’s smaller than the denominator (the number on the bottom). A few examples would be 1/2, 3/11 and 9/10.
In an improper fraction, it’s the opposite. The numerator is larger than the denominator. Some examples: 5/4, 7/3 and 24/11.
Lastly, keep in mind that mixed fractions contain a whole number and a proper fraction, which we can get from an improper fraction since there’s always a whole number in an improper fraction.
In the examples above, we can convert all improper fractions into the mixed fractions 11/4, 21/3 and 22/11.
Multiplying Fractions
The process in multiplying fractions is pretty straightforward: you multiply the top numbers then you multiply the bottom numbers. Let’s see a few examples on how it would work on the different types of fractions.
How to Multiply Proper Fractions
There are 3 simple steps to multiplying proper fractions.
- Step 1: Multiply the numerators with each other
- Step 2: Multiply the denominators with each other
- Step 3: Simplify (if applicable)
For example, we have 1/2 and 4/9. If we multiply these numbers, we’ll have (1 * 4)/(2 * 9).
If we multiply the numerators, we get 4, then if we multiply the denominators, we get 18. Put them together and you have 4/18.
We can further simplify this number, so we’ll end up with 2/9. This is the correct answer.
How to Multiply Improper Fractions
The process with improper fractions is the same as the above. The only difference is that your teacher might require you to simplify the answer further to a mixed number. The steps then become:
- Step 1: Multiply the numerators with each other
- Step 2: Multiply the denominators with each other
- Step 3: Simplify (if applicable)
- Step 4: Turn into a mixed fraction
For example, 3/2 * 6/5 will become (3 * 6)?(2 * 5). Multiplying the top numbers, we get 18; and then multiplying the bottom numbers, we get 10.
Now we have 18/10, which we can simplify to 9/5. We can leave it at that, but if your teacher requires you to convert improper fractions into a series of mixed fractions, we’ll simplify it further to 1 and 4/5.
How to Multiply Mixed Fractions
In case you have mixed fractions, turn them into improper fractions first. Afterward, the process of multiplying mixed numbers is still the same as the above.
- Step 1: Convert mixed fractions into improper fractions
- Step 2: Multiply the numerators with each other
- Step 3: Multiply the denominators with each other
- Step 4: Simplify (if applicable)
- Step 5: Turn into a mixed fraction
Suppose we have 1 and 3/4 * 2 and 1/2. We turn each one into improper fractions first, so now we’ll have 7/4 and 5/2, which we can now multiply using the process above.
(7 * 5)/(4 * 2) becomes 35/8. We can’t simplify this to a smaller number, so we’ll proceed with simplifying it back to a mixed fraction. We’ll get 4 and 3/8.
Dividing Fractions
Dividing has a similar process to multiplying fractions, but we’ll have to do one thing beforehand. This early step helps combat the confusing process of diving fractions.
First, you get the reciprocal of the divisor and then proceed with the problem using the multiplication process.
- Step 1: Get the reciprocal of the denominator
- Step 2: Multiply the numerators with each other
- Step 3: Multiply the denominators with each other
- Step 4: Simplify (if applicable)
Let’s take a look at examples.
How to Divide Proper Fractions
Dividing proper fractions is one of the simplest procedures compared to the other methods on this list. That said, many young students still get confused because it requires multiplication instead of straight up division.
Let’s divide 3/5 by 2/3. In this example, 2/3 is the divisor, the number or fraction that’s on the right side of the equation. Let’s get the reciprocal by inverting the numbers, so now we have 3/2.
Now, let’s multiply it. Note that it ends up as an improper fraction, but it doesn’t matter as the process is still the same.
The equation becomes (3 * 3)/(5 * 2), which then equals to 9/10. You can’t simplify or reduce this number further, so this is the final answer.
How to Divide Improper Fractions
The process doesn’t change when compared to dividing proper fractions. But, let’s give you an example to properly see how it works. Here’s one with both improper fractions.
4/3 / 5/2. What’s the divisor? That’s right – it’s 5/2, so now we’ll get its reciprocal, which is 2/5. Now, we’ll proceed with multiplying these numbers.
(4 * 2)/(3 * 5) is 8/15. Since this is a proper fraction that you can’t simplify further, you don’t have to turn it into a mixed fraction.
How to Divide Mixed Fractions
Like in multiplying mixed numbers, there’s an extra step before you proceed with the process.
- Step 1: Turn mixed fractions into improper fractions
- Step 2: Get the reciprocal of the denominator
- Step 3: Multiply the numerators with each other
- Step 4: Multiply the denominators with each other
- Step 5: Simplify (if applicable)
- Step 6: Turn improper fractions into mixed fractions
Let’s take 6 and 1/2 and 1 and 5/4. Following step 1, this gives us 13/2 and 9/4. Following step 2, 13/2 / 9/4 becomes (13 * 4)/(2 * 9).
Multiply those numbers to get 52/18. We can simplify this to 26/9. As it’s an improper fraction, you’ll have to turn it into a whole number.
The final answer becomes 2 and 8/9.
Multiplying and Dividing Fractions Still Confusing?
Don’t worry! You’ll get it by practicing continuously until multiplying and dividing fractions, no matter the type, becomes second nature to you.
Find online resources that can give you challenges to answer. You can also challenge yourself or ask other people to give you fractions that you can multiply or divide. Then, check if your answer is correct using our online fraction calculator.